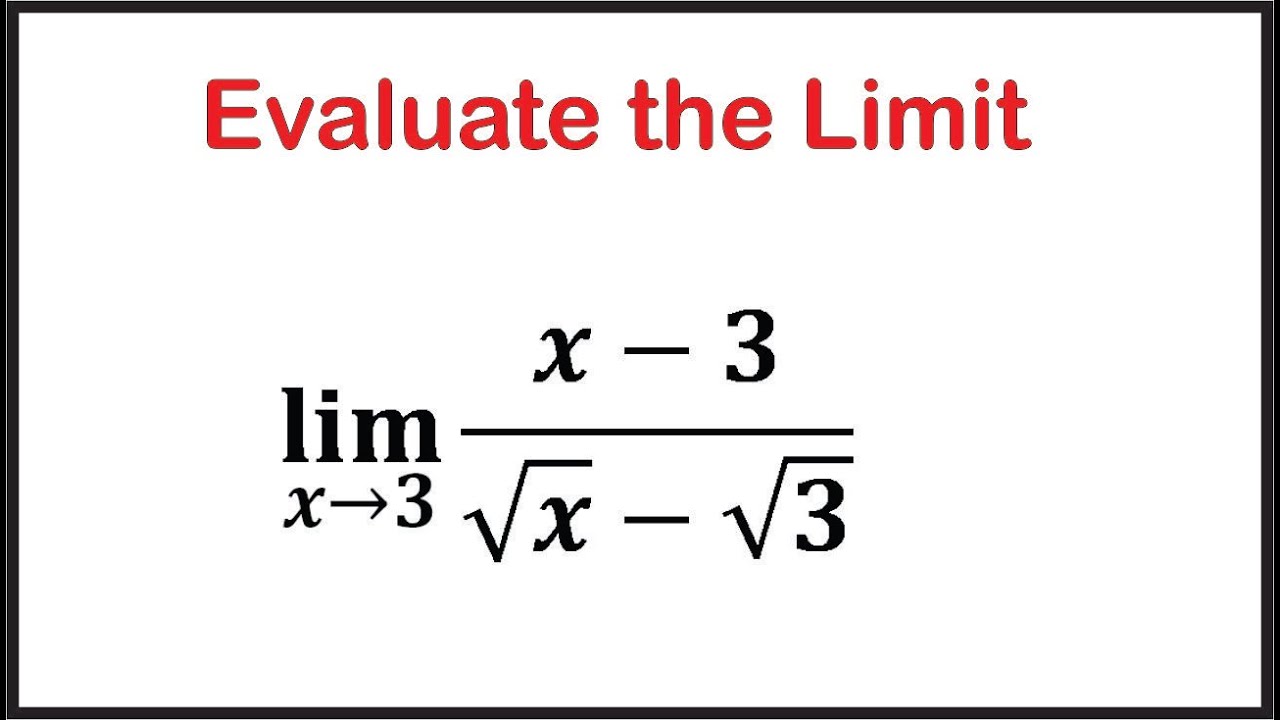
How to compute limits? This is a question that often perplexes students venturing into the fascinating world of calculus. Limits form the foundation of calculus, a branch of mathematics that explores the concepts of change and motion. Understanding how to compute limits is crucial as it opens the door to more complex topics like derivatives and integrals. Whether you're a student tackling your first calculus course or a math enthusiast seeking to deepen your knowledge, mastering the computation of limits is an essential skill.
Calculus may seem intimidating at first, but fear not! With the right guidance and practice, computing limits can become a straightforward and rewarding process. Limits help define and understand the behavior of functions as they approach specific points or infinity. They provide a mathematical way to capture the idea of approaching, getting closer, and even touching, but not necessarily reaching a point. By learning how to compute limits efficiently, you can unravel the mysteries of calculus and gain a deeper appreciation for the beauty of mathematics.
In this comprehensive guide, we will delve into the intricacies of calculating limits. We will explore various techniques and methods, from the basic principles to more advanced approaches, ensuring that you have a solid understanding of the subject. You'll learn how to identify when limits exist, how to handle indeterminate forms, and how to apply these concepts to real-world problems. So, let's embark on this mathematical journey together and discover the art of computing limits!
Table of Contents
- Understanding the Concept of Limits
- The Importance of Limits in Calculus
- Basic Techniques for Computing Limits
- Advanced Techniques for Computing Limits
- Limits at Infinity
- One-Sided Limits
- Indeterminate Forms and L'Hôpital's Rule
- Continuity and Limits
- The Role of Limits in Derivatives
- The Role of Limits in Integrals
- Real-World Applications of Limits
- Common Mistakes to Avoid
- Frequently Asked Questions
- Conclusion
Understanding the Concept of Limits
The concept of limits is central to calculus and provides a way to examine the behavior of functions as they approach a particular point. A limit captures the idea of what a function approaches as the input (or x-value) gets closer and closer to a specific value. It helps us explore the idea of getting infinitesimally close to a point and observing the behavior of the function at that proximity.
Mathematically, a limit is expressed as:
limx→c f(x) = L
This notation indicates that as x approaches c, the function f(x) approaches the value L. It's important to note that the limit doesn't necessarily mean that the function actually reaches the value L at x = c, but rather describes the behavior around that point.
There are several key concepts to grasp when working with limits:
- Approaching from the Left: This is denoted as limx→c⁻ f(x) and describes the limit of a function as x approaches c from values less than c.
- Approaching from the Right: This is denoted as limx→c⁺ f(x) and describes the limit of a function as x approaches c from values greater than c.
- Two-Sided Limit: If both the left-hand and right-hand limits exist and are equal, the two-sided limit exists.
Understanding these foundational concepts is crucial for computing limits effectively. By mastering the notion of limits, you can analyze the behavior of functions near specific points and apply this understanding to a wide range of mathematical problems.
The Importance of Limits in Calculus
Limits are the cornerstone of calculus, serving as the gateway to the study of derivatives and integrals. They provide a mathematical framework for understanding change, continuity, and the behavior of functions. Let's explore why limits are so vital in calculus:
1. Defining Derivatives: The concept of a limit is fundamental in defining derivatives. A derivative represents the rate of change of a function at a given point and is defined as the limit of the average rate of change over an interval as the interval approaches zero. Without limits, the precise calculation of derivatives wouldn't be possible.
2. Determining Continuity: Limits help determine whether a function is continuous at a specific point. A function is continuous if its limit at a point equals the function's value at that point. Continuity is a crucial concept in calculus, forming the basis for many theorems and applications.
3. Integral Calculus: Limits also play a significant role in integral calculus. Definite integrals are defined as the limit of a sum, known as a Riemann sum, as the number of subintervals approaches infinity. Understanding limits is essential for calculating the area under a curve and solving various real-world problems.
The importance of limits extends beyond theoretical mathematics. They have practical applications in fields such as physics, engineering, economics, and computer science. By grasping the concept of limits, you gain a powerful tool for analyzing change and solving complex problems in diverse disciplines.
Basic Techniques for Computing Limits
Computing limits involves a variety of techniques that allow us to evaluate the behavior of functions as they approach specific points. Let's explore some fundamental methods for calculating limits:
1. Direct Substitution: The simplest method for calculating limits is direct substitution. If the function is continuous at the point where the limit is being evaluated, you can directly substitute the value of x into the function to find the limit. However, this method may not work for all functions, especially if it leads to an indeterminate form.
2. Factoring: When direct substitution results in an indeterminate form like 0/0, factoring can be a useful technique. By factoring the numerator and denominator, you may be able to cancel common terms and simplify the expression, allowing for direct substitution.
3. Rationalization: Rationalization is a technique used when dealing with limits involving square roots. By multiplying the numerator and denominator by the conjugate of the expression, you can eliminate the square root and simplify the expression, making it easier to evaluate the limit.
4. Simplification: Simplifying complex expressions can often make it easier to compute limits. This involves using algebraic manipulations to break down the function into more manageable parts, allowing for easier evaluation.
By mastering these basic techniques, you'll be equipped to handle a wide range of limit problems. Practice is key, so be sure to work through various examples to solidify your understanding and build confidence in computing limits.
Advanced Techniques for Computing Limits
While basic techniques are sufficient for many limit problems, some situations require more advanced methods to find solutions. Let's explore some advanced techniques for computing limits:
1. L'Hôpital's Rule: L'Hôpital's Rule is a powerful tool for solving limits that result in indeterminate forms such as 0/0 or ∞/∞. The rule states that if the limit of a function is in an indeterminate form, the limit of the derivatives of the numerator and denominator can be evaluated instead. This method can greatly simplify the process of computing limits.
2. Squeeze Theorem: The Squeeze Theorem is useful when it's challenging to directly evaluate a limit. It involves finding two functions that "squeeze" the target function from above and below, and if the limits of these two functions are equal, the limit of the target function is the same.
3. Series Expansion: In some cases, using series expansion can help evaluate limits, especially when dealing with complex functions. By expressing a function as a power series, you can analyze the behavior of the function as it approaches a specific point, allowing for limit evaluation.
4. Change of Variables: Changing variables can simplify complex expressions, making it easier to compute limits. This technique involves substituting a different variable to transform the expression into a more manageable form.
Advanced techniques require a deeper understanding of calculus concepts, but they provide powerful tools for tackling challenging limit problems. By honing these skills, you'll be well-prepared to handle even the most complex limit computations.
Limits at Infinity
Limits at infinity deal with the behavior of functions as the input variable approaches positive or negative infinity. Understanding how to compute limits at infinity is crucial for analyzing the end behavior of functions and determining asymptotic behavior.
When evaluating limits at infinity, consider the following:
1. Polynomial Functions: For polynomial functions, the behavior as x approaches infinity is determined by the leading term. The degree and coefficient of the leading term dictate whether the function approaches positive or negative infinity or a finite value.
2. Rational Functions: For rational functions, the degree of the numerator and denominator plays a key role in determining the limit at infinity. If the degrees are equal, the limit is the ratio of the leading coefficients. If the denominator's degree is higher, the limit is zero. If the numerator's degree is higher, the limit is infinite.
3. Exponential and Logarithmic Functions: Exponential functions tend to grow rapidly as x approaches infinity, while logarithmic functions grow slowly. Understanding the behavior of these functions is essential for evaluating limits at infinity.
By analyzing the end behavior of functions, you can effectively compute limits at infinity and gain insights into the long-term trends of mathematical functions.
One-Sided Limits
One-sided limits focus on the behavior of a function as it approaches a specific point from one direction only — either from the left or the right. Understanding one-sided limits is crucial for analyzing functions that exhibit different behavior on either side of a point.
Consider the following when evaluating one-sided limits:
1. Left-Hand Limit: The left-hand limit, denoted as limx→c⁻ f(x), describes the limit of a function as x approaches c from values less than c. It's important to examine the behavior of the function exclusively from the left side.
2. Right-Hand Limit: The right-hand limit, denoted as limx→c⁺ f(x), describes the limit of a function as x approaches c from values greater than c. It focuses on the behavior of the function exclusively from the right side.
One-sided limits are often used to determine the existence of a two-sided limit. If both the left-hand and right-hand limits exist and are equal, the two-sided limit exists. One-sided limits are also valuable for analyzing piecewise functions and identifying points of discontinuity.
Indeterminate Forms and L'Hôpital's Rule
Indeterminate forms arise when evaluating limits leads to expressions that don't have an obvious value, such as 0/0, ∞/∞, or 0×∞. L'Hôpital's Rule is a powerful tool for resolving these indeterminate forms and finding the limit.
L'Hôpital's Rule states that if the limit of a function is in an indeterminate form, the limit of the derivatives of the numerator and denominator can be evaluated instead. This rule can greatly simplify the process of computing limits, especially for complex expressions.
Consider the following steps when applying L'Hôpital's Rule:
1. Identify the Indeterminate Form: Verify that the limit results in an indeterminate form such as 0/0 or ∞/∞.
2. Differentiate the Numerator and Denominator: Find the derivatives of the numerator and denominator separately.
3. Evaluate the New Limit: Substitute the original point of interest into the new expression and evaluate the limit.
Keep in mind that L'Hôpital's Rule can be applied multiple times if necessary, but it's essential to ensure that the conditions for the rule are met. This technique is a valuable tool for resolving challenging limit problems and finding meaningful solutions.
Continuity and Limits
Continuity is a fundamental concept in calculus that describes the smoothness and uninterrupted behavior of a function. Understanding the relationship between continuity and limits is essential for analyzing functions and solving calculus problems.
A function is continuous at a point if the following conditions are met:
- The function is defined at the point.
- The limit of the function exists at the point.
- The limit of the function equals the function's value at the point.
Continuity ensures that a function doesn't have any sudden jumps, breaks, or holes at a specific point. It's an important property for analyzing functions and applying theorems like the Intermediate Value Theorem and the Mean Value Theorem.
By understanding the relationship between continuity and limits, you can effectively analyze the behavior of functions, determine points of discontinuity, and solve a wide range of mathematical problems.
The Role of Limits in Derivatives
Derivatives are a cornerstone of calculus, representing the rate of change of a function at a given point. The concept of limits is fundamental in defining and calculating derivatives, as they provide a mathematical framework for understanding instantaneous change.
The derivative of a function f(x) at a point c is defined as:
f'(c) = limh→0 [f(c+h) - f(c)]/h
This definition, known as the limit definition of the derivative, captures the idea of the instantaneous rate of change as the interval approaches zero. It highlights the importance of limits in analyzing the behavior of functions and understanding the concept of differentiation.
By mastering the computation of limits, you can effectively calculate derivatives, analyze the behavior of functions, and solve complex mathematical problems involving change and motion.
The Role of Limits in Integrals
Integrals are another fundamental concept in calculus, representing the accumulation of quantities and the area under a curve. Limits play a crucial role in defining and calculating integrals, as they provide a mathematical framework for understanding the concept of accumulation.
The definite integral of a function f(x) over an interval [a, b] is defined as the limit of a sum:
∫ab f(x) dx = limn→∞ Σ f(xi)Δx
This definition, known as the limit definition of the integral, captures the idea of accumulation as the number of subintervals approaches infinity. It highlights the importance of limits in analyzing the behavior of functions and understanding the concept of integration.
By mastering the computation of limits, you can effectively calculate integrals, analyze the behavior of functions, and solve complex mathematical problems involving accumulation and area.
Real-World Applications of Limits
Limits have practical applications in various fields, including physics, engineering, economics, and computer science. Understanding how to compute limits is essential for solving real-world problems and making informed decisions.
Consider the following examples of real-world applications of limits:
1. Physics: Limits are used to analyze the behavior of physical systems, such as calculating instantaneous velocity and acceleration. They provide a mathematical framework for understanding change and motion in the natural world.
2. Engineering: Limits are used in engineering to design and analyze systems, such as calculating stress and strain in materials. They provide a mathematical framework for understanding the behavior of structures and systems.
3. Economics: Limits are used in economics to analyze the behavior of markets and make informed decisions. They provide a mathematical framework for understanding change and maximizing profits.
4. Computer Science: Limits are used in computer science to analyze algorithms and optimize performance. They provide a mathematical framework for understanding complexity and efficiency.
By understanding the real-world applications of limits, you can apply mathematical concepts to solve complex problems and make informed decisions in various fields.
Common Mistakes to Avoid
When computing limits, it's important to be aware of common mistakes that can lead to incorrect solutions. By understanding these pitfalls, you can avoid errors and find accurate solutions to limit problems.
Consider the following common mistakes to avoid:
1. Failing to Check Continuity: Before using direct substitution, ensure that the function is continuous at the point where the limit is being evaluated. If the function is not continuous, consider other techniques for computing the limit.
2. Ignoring Indeterminate Forms: When evaluating limits, be mindful of indeterminate forms such as 0/0 or ∞/∞. These forms require additional techniques, such as L'Hôpital's Rule, to find meaningful solutions.
3. Overlooking One-Sided Limits: When evaluating limits, consider the behavior of the function from both the left and right sides. One-sided limits can provide valuable insights into the behavior of the function and the existence of a two-sided limit.
4. Misapplying L'Hôpital's Rule: Ensure that the conditions for L'Hôpital's Rule are met before applying it. The rule is only applicable for certain indeterminate forms, and improper use can lead to incorrect solutions.
By being aware of these common mistakes, you can avoid errors and find accurate solutions to limit problems. Practice is key, so be sure to work through various examples to solidify your understanding and build confidence in computing limits.
Frequently Asked Questions
Q1: What is the purpose of computing limits in calculus?
A1: Computing limits in calculus helps us understand the behavior of functions as they approach specific points or infinity. Limits are foundational for defining derivatives and integrals, and they play a crucial role in analyzing change, continuity, and the behavior of functions.
Q2: How do I know if a limit exists?
A2: A limit exists if the left-hand and right-hand limits are equal and finite. Evaluating the behavior of the function from both sides of the point of interest can help determine the existence of a limit.
Q3: What is an indeterminate form, and how do I resolve it?
A3: An indeterminate form is an expression that doesn't have an obvious value, such as 0/0 or ∞/∞. To resolve it, use techniques like L'Hôpital's Rule, factoring, or rationalization to find meaningful solutions.
Q4: How do I compute limits at infinity?
A4: To compute limits at infinity, analyze the end behavior of the function as the input variable approaches positive or negative infinity. Consider the degree and coefficients of polynomial and rational functions, as well as the behavior of exponential and logarithmic functions.
Q5: What is the Squeeze Theorem, and when is it used?
A5: The Squeeze Theorem is used when it's challenging to directly evaluate a limit. It involves finding two functions that squeeze the target function from above and below. If the limits of these two functions are equal, the limit of the target function is the same.
Q6: Can I use L'Hôpital's Rule for all limit problems?
A6: No, L'Hôpital's Rule is only applicable for certain indeterminate forms, such as 0/0 or ∞/∞. Ensure that the conditions for the rule are met before applying it to find meaningful solutions.
Conclusion
Understanding how to compute limits is a crucial skill in calculus, forming the foundation for more advanced topics like derivatives and integrals. By mastering various techniques and methods, from direct substitution to advanced approaches like L'Hôpital's Rule, you'll be well-equipped to tackle a wide range of limit problems.
Limits provide a mathematical framework for analyzing change, continuity, and the behavior of functions. They have practical applications in fields such as physics, engineering, economics, and computer science, making them an essential tool for solving real-world problems.
By practicing and honing your skills in computing limits, you'll gain a deeper appreciation for the beauty of calculus and the power of mathematics. So, embrace the challenge, explore the art of computing limits, and unlock the mysteries of calculus!
Article Recommendations
- Large Living Room Cabinet
- Sherell Ford
- Bi Fold Exterior Patio Doors
- Gta Iv Script Hook
- Cleaning Kenmore Dishwasher
- Eau De Cologne Et Eau De Toilette
- Melissa Torme March
- Cars With Great Audio Systems
- Proofreading Payment
- Solider Costume