How to make a trapezoid? That's a question that might puzzle you at first glance, but it's a fundamental skill if you're diving into geometry, whether for school projects, construction, or creative design. A trapezoid, or trapezium as it's called in some countries, is a four-sided shape with at least one pair of parallel sides. Understanding how to construct this shape is not only essential for students but also for professionals in various fields. In this guide, we will explore the step-by-step process of creating a trapezoid, ensuring that you grasp the concept with ease and precision.
Creating a trapezoid is not just about drawing lines and connecting dots; it's about understanding its properties, dimensions, and the role it plays in different contexts. As you delve into this article, you'll discover the importance of trapezoids in mathematics, architecture, and even art. We'll cover the necessary tools, techniques, and tips, ensuring you have a well-rounded understanding of this geometric figure. So, whether you're a student preparing for a geometry exam or a hobbyist looking to enhance your design skills, this guide is tailored for you.
Moreover, learning how to make a trapezoid offers a deeper appreciation of geometry's beauty and practicality. From the seamless execution of mathematical principles to the artistic flair in design, trapezoids play a vital role. As you read on, you'll encounter detailed instructions, illustrations, and real-world applications that bring this geometric shape to life. Let's embark on this educational journey and master the art of creating trapezoids, enhancing your understanding and appreciation of the world around you.
Table of Contents
- Understanding the Basics of a Trapezoid
- Tools Required for Making a Trapezoid
- Step-by-Step Guide to Constructing a Trapezoid
- Mathematical Properties of a Trapezoid
- Applications of Trapezoids in Real Life
- Drawing a Trapezoid on Paper
- Constructing a Trapezoid Using Software
- Common Mistakes to Avoid When Making a Trapezoid
- Exploring Different Types of Trapezoids
- The Role of Trapezoids in Architecture
- Trapezoids in Art and Design
- Advanced Techniques for Creating Trapezoids
- FAQs About Making a Trapezoid
- Conclusion
Understanding the Basics of a Trapezoid
Before diving into the construction of a trapezoid, it's crucial to understand its basic properties. A trapezoid is a quadrilateral with at least one pair of parallel sides, known as the bases. The non-parallel sides are called the legs. Depending on the specific type of trapezoid, these sides can vary in length and angle. A standard trapezoid has one pair of parallel sides, while an isosceles trapezoid has legs of equal length and base angles that are congruent.
Understanding the angles and sides of a trapezoid is fundamental. The interior angles of any quadrilateral, including trapezoids, sum up to 360 degrees. In an isosceles trapezoid, the base angles are equal, and the diagonals are also equal in length. This symmetry is what makes the isosceles trapezoid special and often easier to work with in design and calculations.
The significance of trapezoids extends beyond basic geometry. They are employed in various practical applications, from calculating areas in construction to designing aesthetically pleasing structures. Understanding the basics of trapezoids lays the foundation for more advanced applications and designs.
Tools Required for Making a Trapezoid
To construct a trapezoid accurately, you'll need a few essential tools. These tools help ensure precision and ease in creating the shape, whether you are drawing it on paper or constructing it in a digital format.
For physical construction on paper or other materials, the following tools are necessary:
- Ruler: A ruler is essential for measuring and drawing straight lines accurately.
- Protractor: This tool helps in measuring and drawing precise angles, which is crucial for ensuring the legs and bases are correctly angled.
- Compass: A compass is useful for drawing arcs and circles, which can assist in ensuring symmetry and equal length of sides when necessary.
- Pencil: A good pencil, preferably with an eraser, allows for making adjustments and corrections easily.
- Graph Paper: Using graph paper can help maintain proportions and ensure the trapezoid is symmetrical.
For digital construction, software tools such as CAD (Computer-Aided Design) programs can be invaluable. These programs allow for precise measurements and easy adjustments, making them ideal for complex designs. Software like AutoCAD, SketchUp, or even basic drawing tools like Microsoft Paint can be used to construct trapezoids digitally with precision.
Having the right tools at your disposal not only facilitates the process but also enhances the accuracy of your trapezoid construction, whether physically or digitally.
Step-by-Step Guide to Constructing a Trapezoid
Constructing a trapezoid involves understanding its properties and applying them to create an accurate shape. Here is a step-by-step guide to help you through the process:
- Identify the Dimensions: Determine the lengths of the bases and the height of the trapezoid. This initial step is crucial as it sets the foundation for the rest of the construction.
- Draw the Base: Using a ruler, draw the longer base of the trapezoid on your chosen surface. Ensure it is straight and measures the correct length as per your dimensions.
- Measure the Height: From a point on the base, use a protractor to draw a perpendicular line representing the height of the trapezoid. This line will help determine the position of the second base.
- Draw the Second Base: At the top of the height line, draw the second base parallel to the first. Use your ruler to ensure it's parallel and measures the correct length.
- Connect the Legs: Finally, connect the ends of the two bases with straight lines to form the legs of the trapezoid. Use a ruler to ensure these lines are straight and meet the bases at the correct angles.
Following these steps will result in a well-constructed trapezoid. It's important to double-check measurements and angles to ensure accuracy, especially if the trapezoid will be used in further calculations or design work.
Mathematical Properties of a Trapezoid
Understanding the mathematical properties of a trapezoid is crucial for both construction and application. These properties include its angles, side lengths, and other measurements that define the shape.
The sum of the interior angles in a trapezoid is always 360 degrees. This is a fundamental property of all quadrilaterals. In an isosceles trapezoid, the base angles are equal, and the sum of the angles on the same side of the trapezoid is 180 degrees. This symmetry can simplify calculations and construction.
The area of a trapezoid can be calculated using the formula:
Area = (Base1 + Base2) × Height / 2
Here, Base1 and Base2 are the lengths of the parallel sides, and the height is the perpendicular distance between them. This formula is essential for determining the space a trapezoid occupies, whether on paper or in physical space.
Another important property is the midsegment or median of the trapezoid, which is a line segment connecting the midpoints of the legs. The length of the midsegment is the average of the lengths of the two bases.
These mathematical properties are not only essential for calculations but also for understanding the structure and balance of trapezoids in various applications.
Applications of Trapezoids in Real Life
Trapezoids are more than just a geometric concept; they have practical applications in various fields. Understanding these applications can provide context and motivation for mastering their construction and properties.
In architecture and construction, trapezoids are often used in the design of roofs, bridges, and other structures. Their unique shape allows for stability and aesthetic appeal. Trapezoidal shapes can distribute weight effectively, making them a popular choice in structural design.
In mathematics and physics, trapezoids are used to model and solve problems involving areas and forces. The simplicity of calculating the area of a trapezoid makes it a useful shape in many analytical applications.
Trapezoids also appear in art and design, where their distinctive shape can be used to create visually interesting compositions. From modern art to graphic design, trapezoids offer a unique aesthetic that can be both functional and decorative.
Understanding the diverse applications of trapezoids can inspire innovative uses and encourage a deeper appreciation of their role in both practical and creative fields.
Drawing a Trapezoid on Paper
Drawing a trapezoid on paper is a practical skill that can be useful in various contexts, from academic assignments to design projects. Here are the steps to draw a trapezoid accurately:
- Prepare Your Tools: Ensure you have a ruler, protractor, pencil, and eraser ready. Use graph paper if available, as it can help maintain proportions.
- Draw the Base: Use the ruler to draw a straight line that will serve as the base of the trapezoid. Ensure it is the desired length.
- Measure the Height: At one end of the base, use the protractor to draw a perpendicular line representing the height of the trapezoid. This line will help position the second base.
- Draw the Second Base: From the top of the height line, draw a parallel line to the first base, ensuring it is the correct length for the second base.
- Connect the Legs: Finally, connect the ends of the two bases with straight lines to form the trapezoid's legs. Use the ruler to ensure the lines are straight and meet the bases correctly.
By following these steps, you can draw a trapezoid accurately and with confidence. Remember to double-check your measurements and angles, as accuracy is crucial in both artistic and technical drawings.
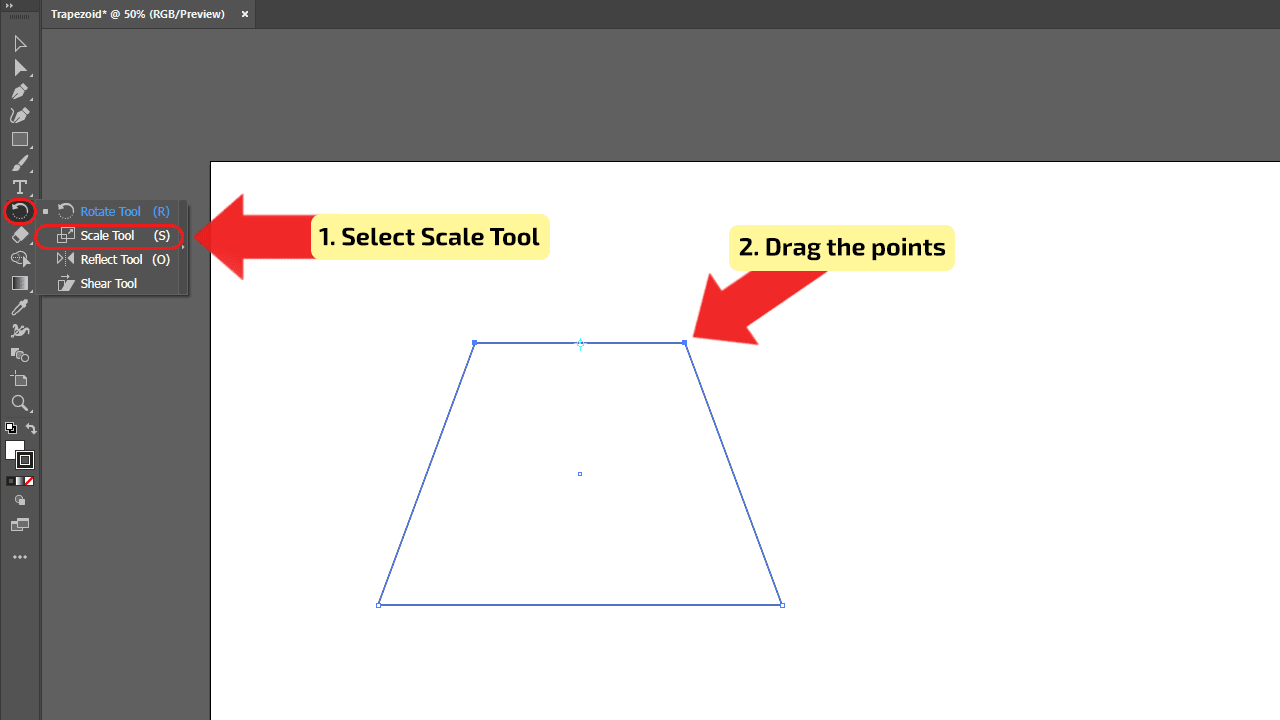
Constructing a Trapezoid Using Software
In today's digital age, constructing a trapezoid using software can offer precision and flexibility. Whether for design, engineering, or educational purposes, digital tools provide a modern approach to geometry.
To construct a trapezoid using software, follow these steps:
- Select Your Software: Choose a suitable software program, such as AutoCAD, SketchUp, or even a basic drawing tool like Microsoft Paint. Each offers different features and levels of precision.
- Create a New Project: Open a new project or document within the software. Set your canvas size and units of measurement according to your needs.
- Draw the Base: Use the software's line tool to draw the first base of the trapezoid. Ensure it is straight and measures the correct length.
- Set the Height: Draw a perpendicular line from one end of the base to represent the trapezoid's height. This line will guide the position of the second base.
- Draw the Second Base: Using the parallel line tool, draw the second base at the top of the height line, ensuring it is parallel to the first base and measures the correct length.
- Complete the Shape: Connect the ends of the two bases with straight lines to form the legs of the trapezoid. Use the software's snapping or alignment features to ensure precision.
Constructing a trapezoid digitally offers advantages such as easy adjustments, precise measurements, and the ability to save and share your work electronically. It's a valuable skill for both students and professionals in the digital age.
Common Mistakes to Avoid When Making a Trapezoid
While constructing a trapezoid, there are common mistakes that can occur, compromising the accuracy and integrity of the shape. Being aware of these pitfalls can help you avoid them and ensure a successful outcome.
Incorrect Measurements: One of the most common mistakes is inaccurate measurements. Ensure you double-check the lengths of the bases and the height before starting your construction.
Improper Angles: Another frequent error is drawing lines at incorrect angles. Use a protractor to measure angles precisely, especially when creating the height and connecting the legs.
Non-Parallel Bases: Failing to ensure that the bases are parallel can result in an incorrect shape. Use a ruler or, in digital applications, alignment tools to maintain parallelism.
Lack of Symmetry: In cases where an isosceles trapezoid is required, symmetry is crucial. Ensure that the legs are of equal length and the base angles are congruent.
Overlooking the Midsegment: The midsegment is an important feature of a trapezoid. Ensure it is accurately positioned and measured, as it can affect the overall balance of the shape.
By being mindful of these common mistakes, you can construct trapezoids with confidence and accuracy, whether on paper or digitally.
Exploring Different Types of Trapezoids
Trapezoids come in various forms, each with unique properties that offer different applications and challenges. Understanding these types can enhance your comprehension and ability to work with trapezoids.
The most common types of trapezoids are:
- Standard Trapezoid: This is the basic form with one pair of parallel sides. The other two sides, or legs, can be of varying lengths and angles.
- Isosceles Trapezoid: An isosceles trapezoid has legs of equal length and base angles that are congruent. This symmetry makes it a popular choice in design and architecture.
- Right Trapezoid: A right trapezoid features one or two right angles. This type is often used in construction and design for its straightforward calculations and applications.
Each type of trapezoid offers distinct properties and uses, from mathematical calculations to artistic design. Understanding these variations allows for versatile applications and a deeper appreciation of trapezoids in both theoretical and practical contexts.
The Role of Trapezoids in Architecture
In architecture, trapezoids play a significant role in both structural and aesthetic design. Their unique shape allows for innovative solutions and creative expression in building projects.
Structurally, trapezoids can be used to distribute weight and provide stability. Their shape is ideal for roof designs, where the slanted sides can effectively channel rainwater and provide an appealing silhouette. In bridges, trapezoidal forms offer strength and balance, allowing for efficient load distribution.
Aesthetically, trapezoids contribute to the visual appeal of buildings and structures. Their distinctive angles and proportions can create dynamic and engaging designs, from modern art installations to historical architectural elements.
Understanding the role of trapezoids in architecture can inspire creative and practical applications, enhancing both the functionality and beauty of buildings and structures.
Trapezoids in Art and Design
In art and design, trapezoids offer a unique and versatile shape that can be used in various creative contexts. Their distinctive form can enhance compositions and add visual interest.
In modern art, trapezoids can be used to create abstract compositions and dynamic shapes. Their angles and proportions offer opportunities for experimentation and innovation, allowing artists to explore new forms and perspectives.
In graphic design, trapezoids can be used to create balanced and engaging layouts. Their shape can guide the viewer's eye and create a sense of movement, making them ideal for advertisements, logos, and other visual media.
Whether in fine art or commercial design, trapezoids offer a versatile and engaging form that can enhance creative expression and visual impact.
Advanced Techniques for Creating Trapezoids
For those looking to explore more advanced techniques in creating trapezoids, there are several methods and tools that can enhance precision and creativity.
Using CAD Software: Computer-Aided Design (CAD) software offers advanced tools for creating precise and detailed trapezoids. These tools allow for easy modifications, accurate measurements, and the ability to save and share designs electronically.
Exploring Descriptive Geometry: Descriptive geometry techniques can be used to create complex and accurate trapezoids. These methods involve understanding the geometric relationships and projections that define the shape, allowing for precise construction.
Experimenting with 3D Modeling: 3D modeling software offers opportunities to create trapezoids in three dimensions, providing a deeper understanding of their properties and applications. These tools are ideal for architects, designers, and engineers looking to explore new possibilities.
By exploring these advanced techniques, you can enhance your skills and understanding of trapezoids, opening up new opportunities for creativity and innovation.
FAQs About Making a Trapezoid
1. What is the easiest way to draw a trapezoid?
The easiest way to draw a trapezoid is to start with a straight base, use a protractor to measure the height, and then draw the second parallel base. Connect the ends with straight lines to complete the shape.
2. Can trapezoids have more than one pair of parallel sides?
No, a trapezoid has only one pair of parallel sides. If a quadrilateral has more than one pair of parallel sides, it is classified as a parallelogram.
3. What is the importance of the midsegment in a trapezoid?
The midsegment, or median, of a trapezoid is a line segment connecting the midpoints of the legs. It is important because it helps in calculating the trapezoid's properties and maintaining symmetry.
4. How do I calculate the area of a trapezoid?
The area of a trapezoid can be calculated using the formula: (Base1 + Base2) × Height / 2. This formula provides the space occupied by the trapezoid.
5. Are trapezoids used in real-life applications?
Yes, trapezoids are used in various real-life applications, including architecture, construction, mathematics, and art. Their unique shape and properties make them versatile and practical.
6. What tools are needed to draw a trapezoid?
To draw a trapezoid, you will need a ruler, protractor, pencil, and eraser. Graph paper can also be useful for maintaining proportions.
Conclusion
Understanding how to make a trapezoid is a valuable skill that extends beyond the classroom. Whether you are a student, architect, artist, or enthusiast, mastering the construction and properties of trapezoids opens up a world of possibilities. From practical applications in construction and design to creative expressions in art and geometry, trapezoids offer a unique blend of functionality and aesthetics.
By following the detailed steps and guidelines provided in this comprehensive guide, you can construct accurate and symmetrical trapezoids with confidence. Whether on paper or digitally, the techniques and insights shared here will enhance your understanding and ability to work with this fascinating geometric shape.
In conclusion, trapezoids are more than just a mathematical concept; they are a gateway to creativity, innovation, and practical application. Embrace the challenge of making trapezoids, and discover the beauty and utility they bring to the world around you.
Article Recommendations
- Margot Robbie Weight Gain
- Evergreen Bushes And Shrubs
- Vegan Restaurants In Sugar Land
- Morgan Kay Beamer
- Encroachment Easement
- Goldman Sachs Pwm Associate Salary
- Quality Metal Detectors
- Semi Gloss Polyurethane
- Reflex Compound Bow
- Evgo Charge Rates