What is 2/5 as a percentage? This question might seem simple, yet it opens up a world of understanding about fractions, percentages, and their applications in everyday life. Fractions and percentages are mathematical concepts that we encounter frequently, whether in our daily shopping, calculating discounts, or even while cooking. Understanding how to convert a fraction like 2/5 into a percentage is not just an academic exercise; it's a practical skill that enhances our ability to make informed decisions.
In this comprehensive guide, we delve into the concept of converting fractions to percentages using the example of 2/5. We will explore the mathematical principles behind this conversion, the step-by-step process of doing so, and its significance in various real-world scenarios. By the end of this article, you will not only know what 2/5 as a percentage is but also appreciate the broader context of its application.
Our exploration will cover various aspects of this transformation, from the historical origins of percentages to their contemporary uses in different fields. We'll also answer frequently asked questions to clear up any lingering doubts. Whether you're a student, a professional, or simply curious, join us as we unravel the mysteries of what converting 2/5 to a percentage entails.
Table of Contents
- Understanding Fractions and Percentages
- The Concept of Fractions
- The Role of Percentages
- The Mathematical Process of Conversion
- Step-by-Step Conversion of 2/5 to a Percentage
- Practical Applications of Percentages
- Historical Background of Percentages
- Common Misunderstandings
- Real-World Examples
- The Importance of Percentages in Daily Life
- The Impact of Percentages in Business
- The Significance of Percentages in Education
- Percentages in Science and Technology
- FAQs
- Conclusion
Understanding Fractions and Percentages
Before diving into the specifics of converting 2/5 to a percentage, it's crucial to understand the fundamental concepts of fractions and percentages. These mathematical tools are not only ubiquitous in academic settings but also in everyday experiences. A fraction, such as 2/5, represents a part of a whole. It is composed of a numerator and a denominator, indicating how many parts of a specific size are considered.
On the other hand, a percentage is a mathematical expression that denotes a number or ratio as a fraction of 100. The term "percent" comes from the Latin words "per centum," meaning "by the hundred." The percentage offers a universal way to express proportions, making comparisons easier across different contexts.
The Concept of Fractions
Fractions are one of the first mathematical concepts taught in school, and for a good reason. They provide a way to express numbers that are not whole, which is essential for a more nuanced understanding of mathematics. A fraction consists of a numerator and a denominator, where the numerator indicates the number of equal parts being considered, and the denominator specifies how many of those parts make up a whole.
For example, in the fraction 2/5, the numerator is 2, and the denominator is 5. This fraction indicates that 2 parts are taken out of a total of 5 parts. Fractions can represent quantities less than, equal to, or greater than one, depending on the relative sizes of the numerator and denominator.
The Role of Percentages
Percentages play a vital role in various aspects of life, including finance, education, business, and science. They provide a convenient way to compare different quantities without ambiguity. When a number is expressed as a percentage, it gives a clear idea of its size relative to a whole.
For instance, saying that 2/5 is equivalent to 40% means that 2 is 40% of 5. This expression simplifies the comparison and understanding of data, especially when dealing with large sets of numbers or when precision is necessary.
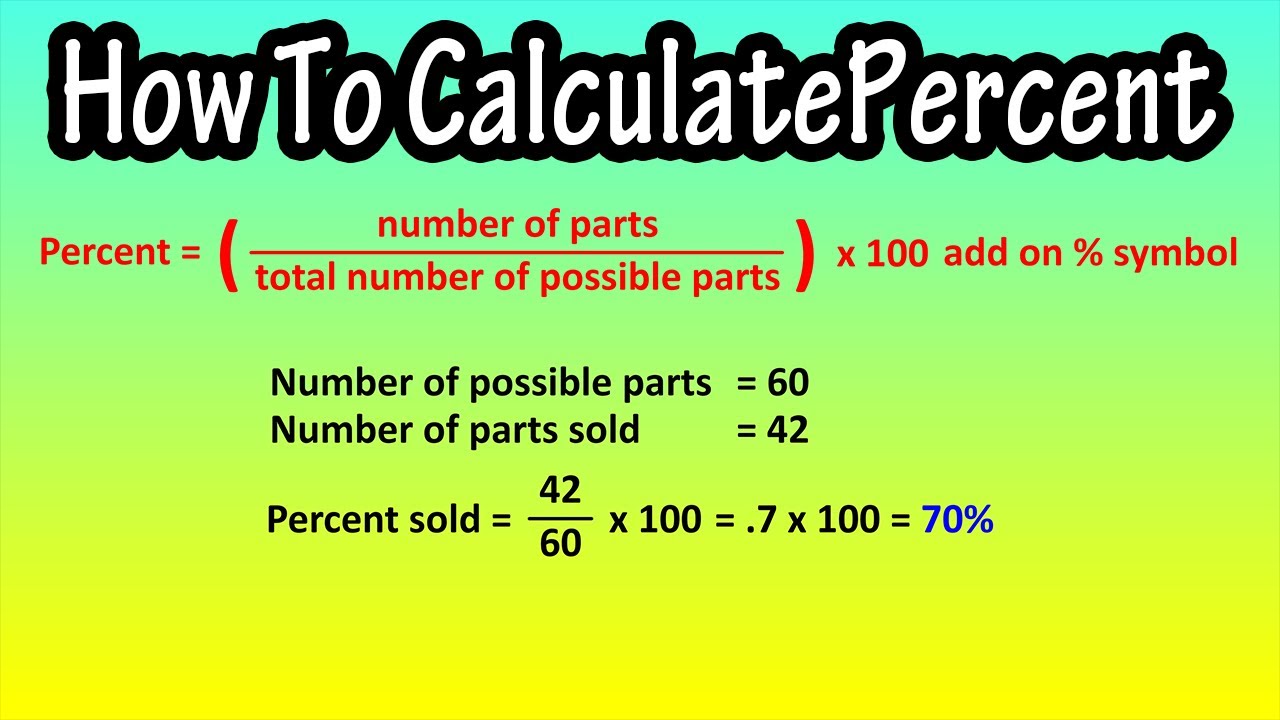
The Mathematical Process of Conversion
Converting fractions to percentages is a straightforward mathematical process that involves a few simple calculations. Understanding this conversion is essential for anyone dealing with data, statistics, or any field that requires precise representation of numbers.
The basic formula for converting a fraction to a percentage is to divide the numerator by the denominator and then multiply the result by 100. This process converts the fraction into a form that is easily understood and compared with other numbers.
Step-by-Step Conversion of 2/5 to a Percentage
Let's walk through the conversion of 2/5 to a percentage using the formula mentioned above. This step-by-step guide will demystify the process and provide a clear pathway for similar conversions.
- Start by dividing 2 by 5. This calculation gives 0.4.
- Next, multiply 0.4 by 100 to convert it into a percentage. The result is 40.
- Therefore, 2/5 as a percentage is 40%.
This simple mathematical process can be applied to any fraction to find its percentage equivalent, making it a valuable skill for students and professionals alike.
Practical Applications of Percentages
Percentages are not just theoretical constructs; they have numerous practical applications. From calculating discounts to understanding statistical data, percentages are indispensable in everyday life. They are used in finance to determine interest rates, in education to calculate grades, and in science to express concentrations.
Understanding how to convert fractions like 2/5 into percentages is crucial for anyone looking to navigate these various fields effectively. Mastery of this skill enhances analytical abilities and improves decision-making processes.
Historical Background of Percentages
The concept of percentages has a rich history that dates back to ancient civilizations. The use of fractions and percentages can be traced to ancient Egypt and Babylon, where they were used in trade and taxation. Over time, the concept evolved, and percentages became a standard measure in various fields, including mathematics, business, and science.
The historical development of percentages highlights their significance and adaptability across different cultures and eras. Today, percentages are an integral part of our numerical language, essential for effective communication and interpretation of quantitative information.
Common Misunderstandings
Despite their widespread use, percentages can sometimes be misunderstood or misinterpreted. One common misconception is that percentages are only applicable to whole numbers. In reality, percentages can represent any ratio, whether whole or fractional.
Another misunderstanding is the assumption that a higher percentage always indicates a better outcome. While percentages provide valuable insights, they must be interpreted in context to avoid misleading conclusions.
Real-World Examples
To further illustrate the importance and application of percentages, let's explore some real-world examples. Whether in finance, education, or science, percentages offer a clear and concise way to express numerical data.
The Importance of Percentages in Daily Life
In daily life, percentages are used in various contexts, from calculating tips at a restaurant to understanding nutritional information on food labels. They provide a simple and effective way to make comparisons and informed choices.
For instance, when shopping, understanding the percentage difference in prices or discounts can lead to better purchasing decisions. Similarly, knowing the percentage of nutrients in food helps in maintaining a balanced diet.
The Impact of Percentages in Business
In the business world, percentages are crucial for financial analysis, budgeting, and forecasting. They help businesses understand their performance, set realistic goals, and make strategic decisions.
For example, a company may use percentage growth in sales to measure its success over time. Similarly, understanding profit margins as percentages allows businesses to assess their profitability effectively.
The Significance of Percentages in Education
In education, percentages are often used to evaluate student performance and academic achievement. Grades are typically expressed as percentages, providing a standardized way to assess and compare student performance.
This use of percentages helps educators identify areas where students excel or need improvement, enabling targeted interventions and support.
Percentages in Science and Technology
In science and technology, percentages are used to express concentrations, efficiencies, and probabilities. They provide a precise and universally understood way to present data, essential for scientific research and technological development.
For instance, in chemistry, the concentration of a solution is often expressed as a percentage, allowing scientists to communicate results clearly and effectively.
FAQs
Q1: What is 2/5 as a percentage?
A1: 2/5 as a percentage is 40%. This conversion is achieved by dividing 2 by 5 to get 0.4 and then multiplying by 100.
Q2: Why are percentages important in daily life?
A2: Percentages are important because they provide a clear way to express and compare values, making it easier to understand data, make informed decisions, and communicate effectively.
Q3: How do percentages help in business?
A3: In business, percentages are used to measure growth, profitability, and performance. They help in analyzing financial data, setting goals, and making strategic decisions.
Q4: Can percentages be greater than 100%?
A4: Yes, percentages can be greater than 100%. This typically occurs when comparing quantities where the part exceeds the whole, such as in cases of profit or growth.
Q5: How do I convert a percentage back to a fraction?
A5: To convert a percentage back to a fraction, divide the percentage by 100 and simplify if necessary. For example, 40% becomes 40/100, which simplifies to 2/5.
Q6: Are there limitations to using percentages?
A6: While percentages are a powerful tool, they must be used wisely. They can sometimes be misleading if not interpreted correctly, especially when context and absolute values are ignored.
Conclusion
Understanding what 2/5 as a percentage is and how to convert fractions to percentages is a fundamental mathematical skill. This knowledge not only enhances one's ability to navigate everyday situations but also provides a foundation for more complex mathematical concepts and applications. Whether in finance, business, education, or science, percentages are an essential part of our numerical language, bridging the gap between raw data and meaningful insights. As we have explored in this guide, the conversion of 2/5 to a percentage is just one example of how mathematics can be applied to simplify and interpret the world around us.
Article Recommendations
- How Far Is Jacksonville From West Palm Beach
- Sherell Ford
- Ui For Apache Kafka Value Filter
- Mexican Pot Luck
- Free Attractions In Niagara Falls
- Thyronorm 50mcg
- Freddie Prinze Jr Jessica Biel
- Emo In Thong
- Eau De Cologne Et Eau De Toilette
- Melting Temperature Of Wax