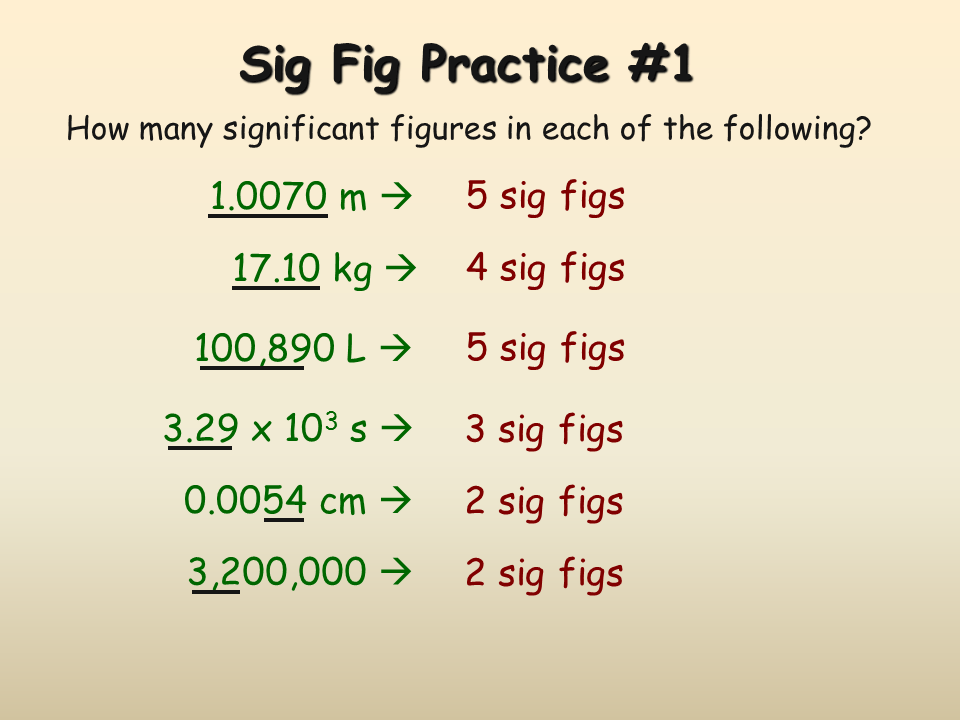
In the world of science and mathematics, precision is key. When dealing with measurements, calculations, and data representation, the concept of significant figures (sig figs) plays a crucial role. Understanding how to determine the appropriate number of significant figures to use can greatly enhance the accuracy and reliability of your work. Whether you're a student, a scientist, or simply someone who enjoys exploring the world of numbers, knowing how to navigate significant figures is essential for clear communication and meaningful analysis.
Significant figures are the digits in a number that contribute to its precision. This includes all non-zero digits, zeros between significant digits, and trailing zeros in a decimal number. However, the rules governing significant figures can be complex, leading many to wonder: how do you know how many sig figs to use? This article will explore the principles behind significant figures, their importance in scientific calculations, and the common rules that govern their use.
As we delve deeper into the topic, we’ll address some common questions, providing clarity on how to approach significant figures in various scenarios. From understanding the foundational concepts to applying them in real-world situations, this guide will equip you with the knowledge you need to confidently determine how many sig figs to use in your measurements and calculations.
What Are Significant Figures?
Significant figures are the digits in a number that provide meaningful information about its precision. They help convey the certainty of a measurement. The significance of a figure depends on its position in the number and whether it contributes to the overall value. Here’s a breakdown of what constitutes significant figures:
- All non-zero digits are always significant.
- Any zeros between significant digits are significant.
- Leading zeros, or zeros before the first non-zero digit, are not significant.
- Trailing zeros in a decimal number are significant.
- Trailing zeros in a whole number without a decimal point are not significant unless specified by a bar or decimal point.
Why Are Significant Figures Important?
Understanding how to determine how many sig figs to use is important for several reasons:
- Accuracy: They help convey the precision of measurements, which is vital in scientific experiments and data analysis.
- Communication: Using the correct number of sig figs ensures that the information is interpreted correctly by others.
- Consistency: Applying significant figures consistently leads to more reliable results in calculations and comparisons.
How Do You Know How Many Sig Figs to Use in Calculations?
When performing calculations involving multiple measurements, the number of significant figures in the final result is determined by the measurement with the least number of significant figures. This is critical to maintain the integrity of the data. Here’s how to apply this principle:
- For addition and subtraction, the result should have the same number of decimal places as the measurement with the least decimal places.
- For multiplication and division, the result should have the same number of significant figures as the measurement with the least significant figures.
What Are Common Rules for Significant Figures?
To confidently determine how do you know how many sig figs to use, familiarize yourself with these common rules:
- Non-Zero Digits: Always significant.
- Leading Zeros: Never significant.
- Captive Zeros: Always significant.
- Trailing Zeros: Significant only in a decimal context.
How Do You Round to the Correct Number of Sig Figs?
Rounding to the appropriate number of significant figures is a critical skill. Here are the steps to follow:
- Identify the last significant figure you want to keep.
- Look at the digit immediately to the right of this figure.
- If that digit is 5 or greater, round up the last significant figure; if it’s less than 5, leave it unchanged.
For example, if you have the number 0.00456 and you want to round it to three significant figures, you would look at the third digit (4) and round up, resulting in 0.00456 becoming 0.00456.
What Are Some Examples of Using Significant Figures?
Let’s explore some examples to illustrate how to determine how many sig figs to use:
- Example 1: If you measure a length as 12.30 cm, it has four significant figures.
- Example 2: A measurement of 0.004500 has four significant figures due to the trailing zeros.
- Example 3: When adding 12.11 (two decimal places) and 0.3 (one decimal place), the result should be rounded to 12.41, maintaining the correct decimal places.
How Do You Know How Many Sig Figs to Use in Scientific Notation?
Scientific notation is a useful way to express very large or very small numbers while maintaining significant figures. In scientific notation, only the digits in the coefficient (the part before the exponent) are counted as significant figures. For instance, in the number 6.022 x 1023, the coefficient 6.022 has four significant figures.
How to Practice Using Significant Figures?
To become proficient in using significant figures, consider the following practice methods:
- Work on practice problems from textbooks or online resources.
- Engage in lab experiments and pay attention to the precision of your measurements.
- Seek feedback from teachers or peers on your calculations involving significant figures.
By consistently applying these rules and concepts, you will develop a strong understanding of how do you know how many sig figs to use in various scenarios.
Conclusion: Mastering Significant Figures
In conclusion, understanding significant figures is essential for anyone working with measurements and calculations. Knowing how do you know how many sig figs to use allows for the precise representation of data and enhances the reliability of scientific communication. By mastering the rules and practicing regularly, you can ensure that your work reflects the appropriate level of accuracy and professionalism. Whether you’re conducting experiments, analyzing data, or simply enjoying the world of numbers, significant figures will be an invaluable tool in your mathematical toolkit.
Article Recommendations
- Glycemic Index Tomato Sauce
- Kobe Thai
- Cartel Murder Photos
- How To Use Rabbitfx
- Price Tag Details
- Zhang Xueying
- Brown Tweed Suit Wedding
- Elasticized Belt
- Ixora Maui Yellow
- Gotlet