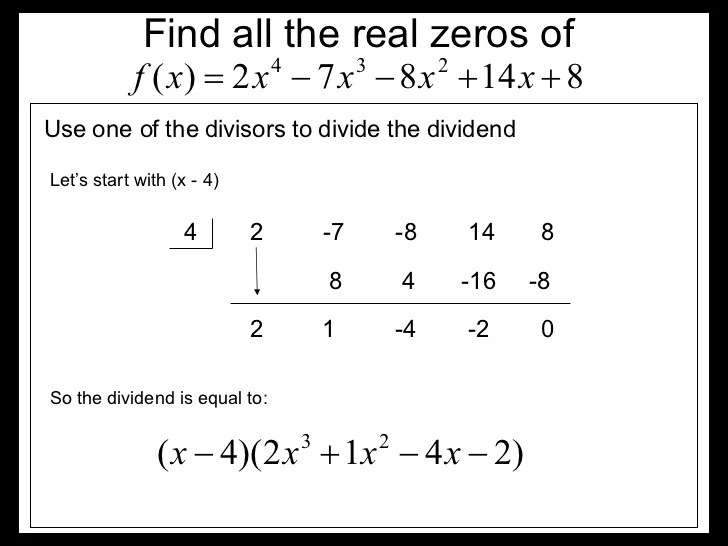
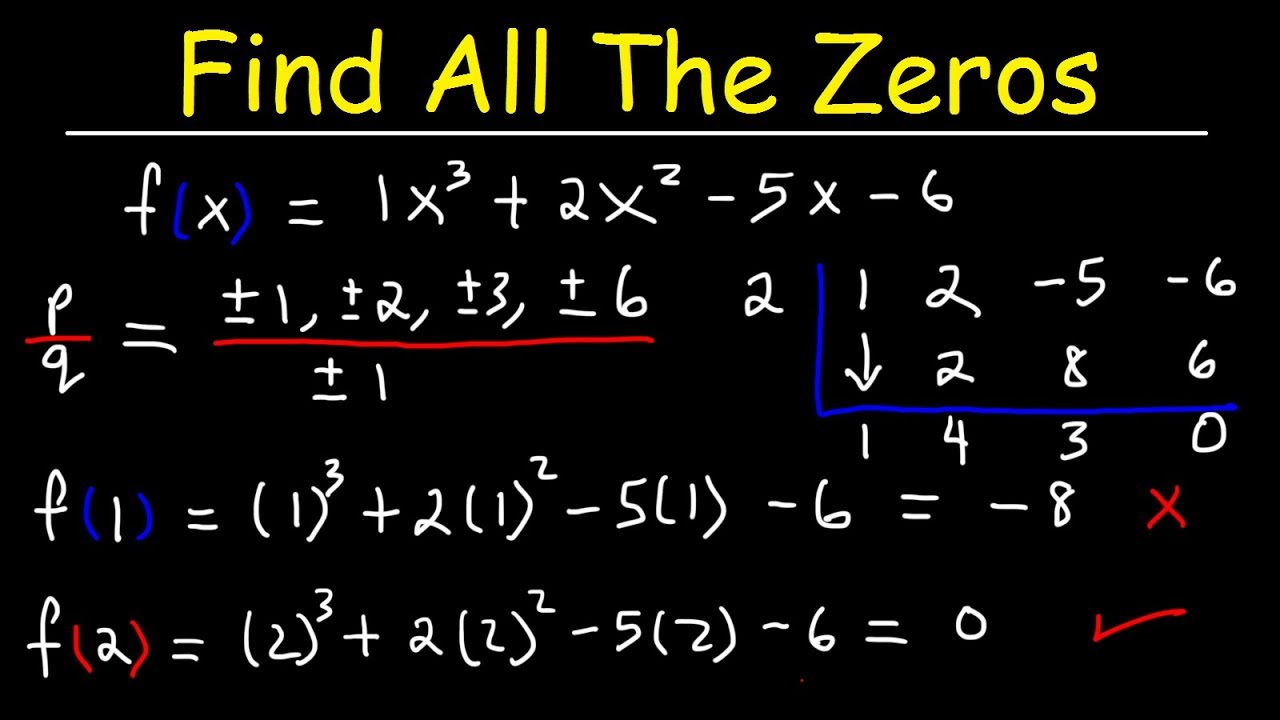
Understanding the concept of real zeros in functions is a foundational skill in mathematics that has far-reaching implications in various fields. Whether you are a student embarking on your mathematical journey or a seasoned professional revisiting the fundamentals, mastering this skill can significantly enhance your analytical abilities. Real zeros, or roots, of a function are the values of the variable for which the function's output is zero. Finding these zeros is crucial in understanding the behavior of functions and solving equations.
In this article, we will explore the various methods and techniques for identifying the real zeros of a function. From graphical methods to algebraic techniques, we will cover a range of approaches that will equip you with the necessary tools to tackle any function. Each method has its own strengths and weaknesses, and understanding when to use each can be the key to successful problem-solving.
Additionally, we will address common questions and challenges that arise when learning how to find the real zeros of a function. By the end of this article, you will be well-prepared to tackle any function and identify its real zeros with confidence and precision.
What Are Real Zeros of a Function?
Real zeros of a function refer to the points at which the function intersects the x-axis. In other words, if you were to graph the function, the real zeros would be the x-coordinates where the output of the function equals zero. These zeros can be classified into different types:
- Single Zeros: A zero that occurs only once.
- Multiple Zeros: A zero that occurs more than once.
- Complex Zeros: Zeros that have imaginary parts, which are not counted when identifying real zeros.
Why Is It Important to Find Real Zeros?
Identifying real zeros is crucial for several reasons:
- Graphical Analysis: Understanding where a function crosses the x-axis helps in sketching the graph accurately.
- Root Finding: Real zeros are essential for solving equations and inequalities.
- Application in Calculus: Real zeros play a significant role in optimization problems and finding local maxima and minima.
How to Find the Real Zeros of a Function Using Graphing?
Graphing is one of the simplest and most intuitive methods for finding real zeros. To graph a function:
- Choose a range for the x-values.
- Calculate the corresponding y-values for each x-value.
- Plot the points on a graph.
- Look for the points where the graph intersects the x-axis.
This method provides a visual representation of the function and allows you to estimate the real zeros. However, it may not always provide exact values, especially for complex functions.
What Are Some Algebraic Methods for Finding Real Zeros?
Algebraic methods are essential for finding exact zeros of a function. Here are some common techniques:
- Factoring: Expressing the function as a product of its factors can help identify zeros.
- Using the Quadratic Formula: For quadratic functions, the formula can directly give you the real zeros.
- Synthetic Division: This method is useful for testing possible rational zeros.
Can You Use the Rational Root Theorem?
The Rational Root Theorem states that any rational solution of a polynomial equation can be expressed as a fraction of the factors of the constant term and the leading coefficient. This theorem can guide you in testing possible rational zeros systematically.
How to Employ Numerical Methods to Find Real Zeros?
Numerical methods are particularly useful when dealing with complex functions where analytical solutions are challenging. Some common numerical techniques include:
- Bisection Method: This method involves repeatedly bisecting an interval and selecting subintervals in which a root must lie.
- Newton's Method: An iterative technique that uses tangents to converge on a zero.
- Secant Method: Similar to Newton's method but does not require the calculation of derivatives.
What Role Do Derivatives Play in Finding Real Zeros?
Derivatives can provide insight into the behavior of a function near its zeros. By analyzing the first derivative, you can determine if a zero is a turning point or if the function crosses the x-axis. This information can help you refine your search for real zeros.
How to Verify Real Zeros Once Found?
Once you have identified potential real zeros, it is crucial to verify them by substituting the values back into the original function. If the output is zero, you have found a valid real zero. Additionally, you can use synthetic division or factoring to ensure that the zeros are correct.
What Are Some Common Challenges When Finding Real Zeros?
Many learners face challenges when trying to find real zeros, such as:
- Identifying the right method for a specific function.
- Underestimating the complexity of the function.
- Misinterpreting the results from graphical or numerical methods.
By understanding these challenges, you can adopt a more strategic approach when learning how to find the real zeros of a function.
Conclusion: Embracing the Journey of Finding Real Zeros
Finding the real zeros of a function is not just a mathematical exercise; it is a skill that fosters critical thinking and problem-solving abilities. By mastering various methods—be it graphical, algebraic, or numerical—you equip yourself with valuable tools that can be applied in numerous situations. Remember, practice is key, and with time, you will become proficient in identifying real zeros with confidence.
As you embark on this journey of discovery, keep an open mind and be willing to explore different approaches. Each function presents a unique challenge, and with each zero you find, you enhance your understanding of mathematics as a whole.
Article Recommendations
- Bi Fold Exterior Patio Doors
- Horny In Sign Language
- Drinking Ambien
- Elasticized Belt
- G3 Case
- Semi Gloss Polyurethane
- Evergreen Bushes And Shrubs
- Robbi Morgan
- Ap Precalculus Unit 3 Review
- Tornado Pro 4 Jacket