In the realm of calculus, integration is a fundamental concept that allows us to calculate the area under curves, among other applications. Among the various integration techniques, the exponential integration rules stand out due to their unique properties and applications in solving complex mathematical problems. These rules are essential for students, educators, and professionals who frequently deal with mathematical modeling and analysis. Understanding these rules not only enhances one’s calculus skills but also opens up a world of possibilities in fields such as physics, engineering, and economics.
The significance of exponential integration rules extends beyond mere academic interest. They provide a systematic approach to solving integrals involving exponential functions, which appear in many real-world scenarios—from population growth models to radioactive decay. Mastering these rules can facilitate quicker problem-solving and a deeper comprehension of various mathematical concepts, thereby fostering a more robust mathematical foundation. Moreover, as we delve into the intricacies of these rules, we will uncover their relevance in both theoretical and applied mathematics.
As we navigate through this article, we will explore the different aspects of exponential integration rules, including their derivation, applications, and common pitfalls. This comprehensive guide aims to clarify any confusion surrounding these rules while providing practical examples that illustrate their effectiveness. Whether you are a student preparing for an exam or a professional seeking to refresh your knowledge, this guide is tailored to meet your needs.
What Are Exponential Integration Rules?
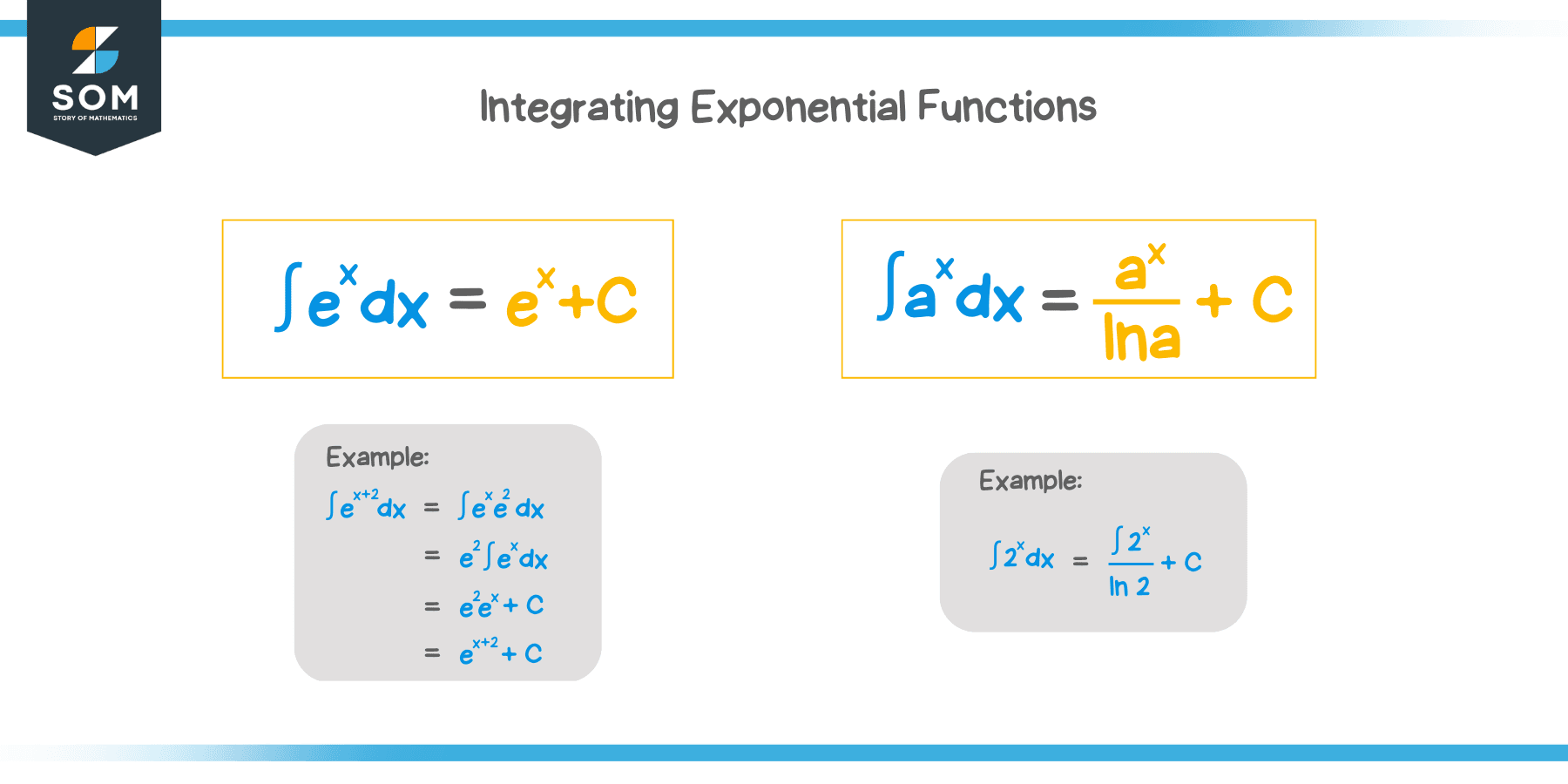
Exponential integration rules refer to specific techniques used to integrate functions involving exponentials, particularly those of the form e^x or a^x, where 'e' is the base of the natural logarithm and 'a' is a constant. These rules are derived from the fundamental properties of exponential functions and can simplify the process of finding definite and indefinite integrals.
Why Are Exponential Integration Rules Important?
Understanding exponential integration rules is crucial for several reasons:
- Application in Various Fields: Exponential functions are prevalent in various disciplines, including biology, economics, and physics.
- Facilitating Problem-Solving: These rules offer efficient methods for tackling integrals that would otherwise be complex and time-consuming.
- Foundation for Advanced Concepts: Mastery of these rules lays the groundwork for more advanced topics in calculus and differential equations.
How Do Exponential Integration Rules Work?
The basic principle underlying exponential integration rules is the relationship between differentiation and integration of exponential functions. For instance, the integral of e^x with respect to x is simply e^x plus a constant of integration (C), a property that holds true for any constant multiplier. This simplicity is what makes exponential functions unique and often easier to integrate than polynomial or trigonometric functions.
What Are Some Common Exponential Integration Rules?
Here are some fundamental exponential integration rules you should familiarize yourself with:
- Rule 1: ∫e^(ax) dx = (1/a)e^(ax) + C, where 'a' is a constant.
- Rule 2: ∫a^(bx) dx = (1/(b ln(a))) a^(bx) + C, where 'a' > 0 and 'a' ≠ 1.
- Rule 3: ∫e^(f(x)) f'(x) dx = e^(f(x)) + C, where f(x) is a differentiable function.
How Can Exponential Integration Rules Be Applied?
The applications of exponential integration rules are vast and varied. Here are a few examples:
- Population Growth Models: In biology, exponential functions are often used to model population growth, where the rate of growth is proportional to the current population size.
- Radioactive Decay: The decay of radioactive substances can be modeled using exponential functions, which can be integrated to determine the remaining quantity over time.
- Financial Calculations: Exponential functions are used in finance to calculate compound interest and investment growth over time.
What Are Some Common Mistakes in Using Exponential Integration Rules?
While the exponential integration rules are straightforward, there are common pitfalls to avoid:
- Misapplying the Constants: Ensure that any constants multiplied with the exponential function are correctly accounted for in the integration process.
- Ignoring the Constant of Integration: Always remember to include the constant of integration (C) unless solving a definite integral.
- Confusing Different Base Functions: Be cautious when working with different bases, as the rules differ slightly depending on whether you are dealing with natural exponentials or other bases.
Conclusion: Mastering Exponential Integration Rules
Mastering the exponential integration rules is an invaluable skill for anyone involved in mathematics or its applications. By understanding how these rules function and practicing their application, you can significantly enhance your problem-solving abilities and mathematical confidence. The exponential integration rules are not merely theoretical constructs, but practical tools that can simplify complex integrals and facilitate a deeper understanding of the natural world. Whether you are a student, educator, or professional, investing time in mastering these rules will undoubtedly pay off in your mathematical journey.
Article Recommendations
- Gta Iv Script Hook
- Brown Tweed Suit Wedding
- Lisa Raye Height
- Glycemic Index Tomato Sauce
- Quality Metal Detectors
- Melting Temperature Of Wax
- Sarah Lahbati Starstruck
- Horny In Sign Language
- Encroachment Easement
- Evgo Charge Rates