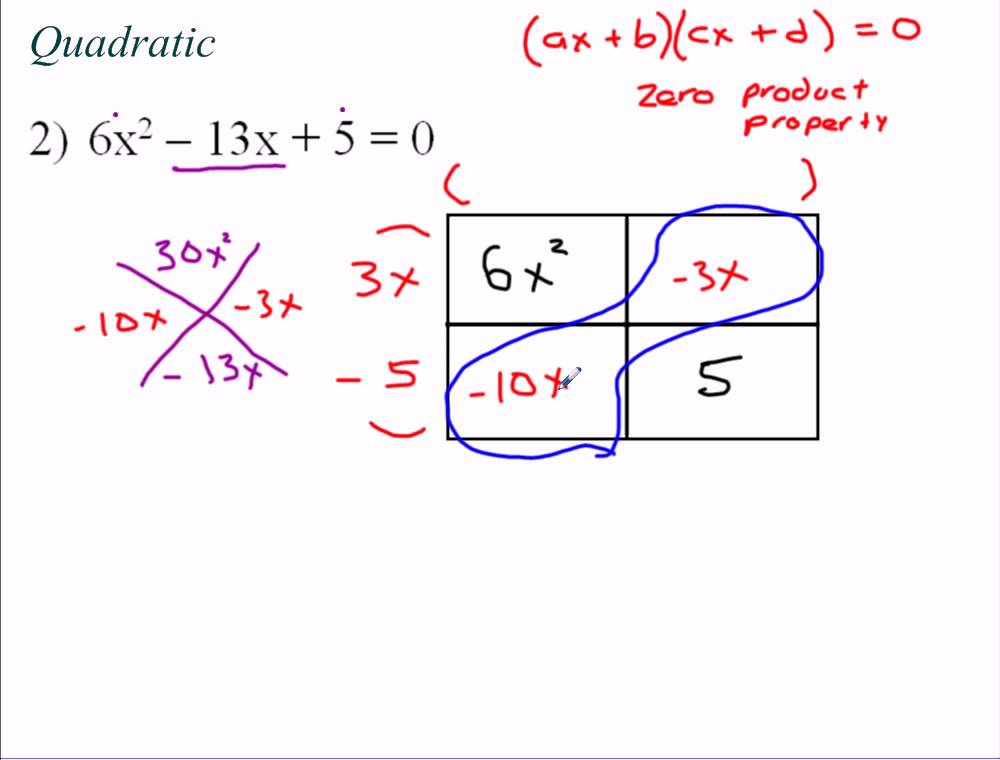
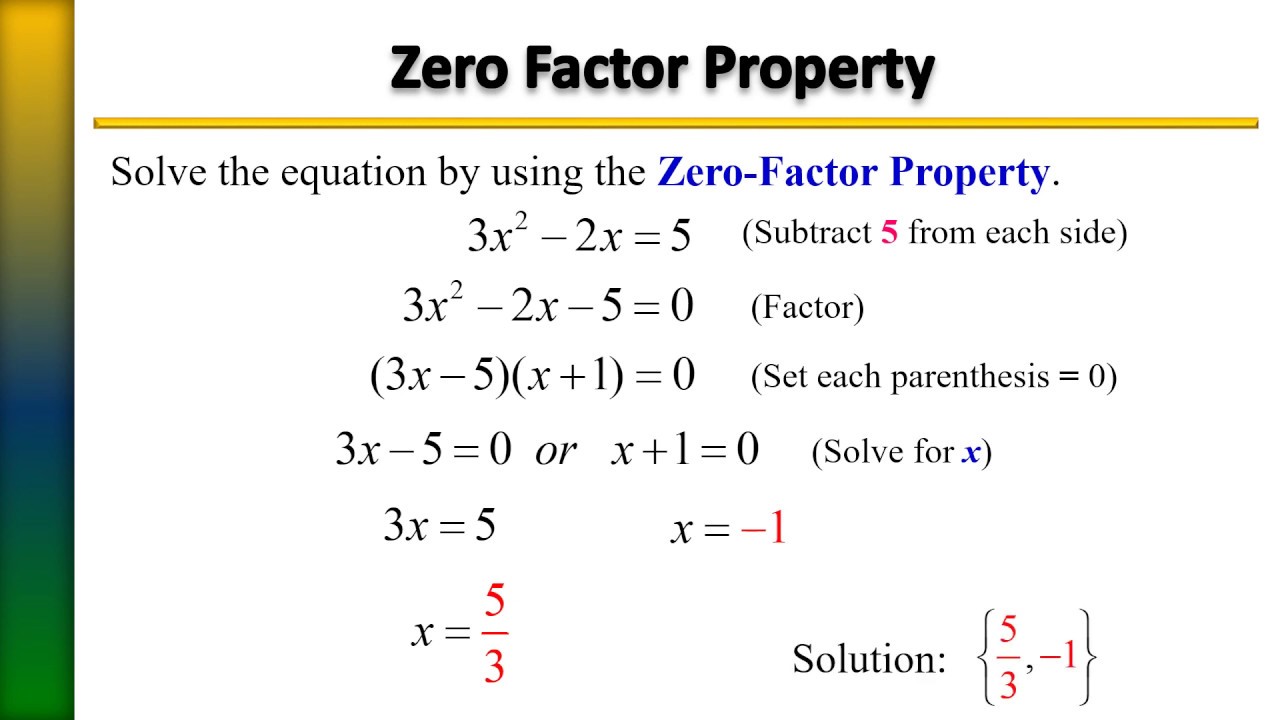
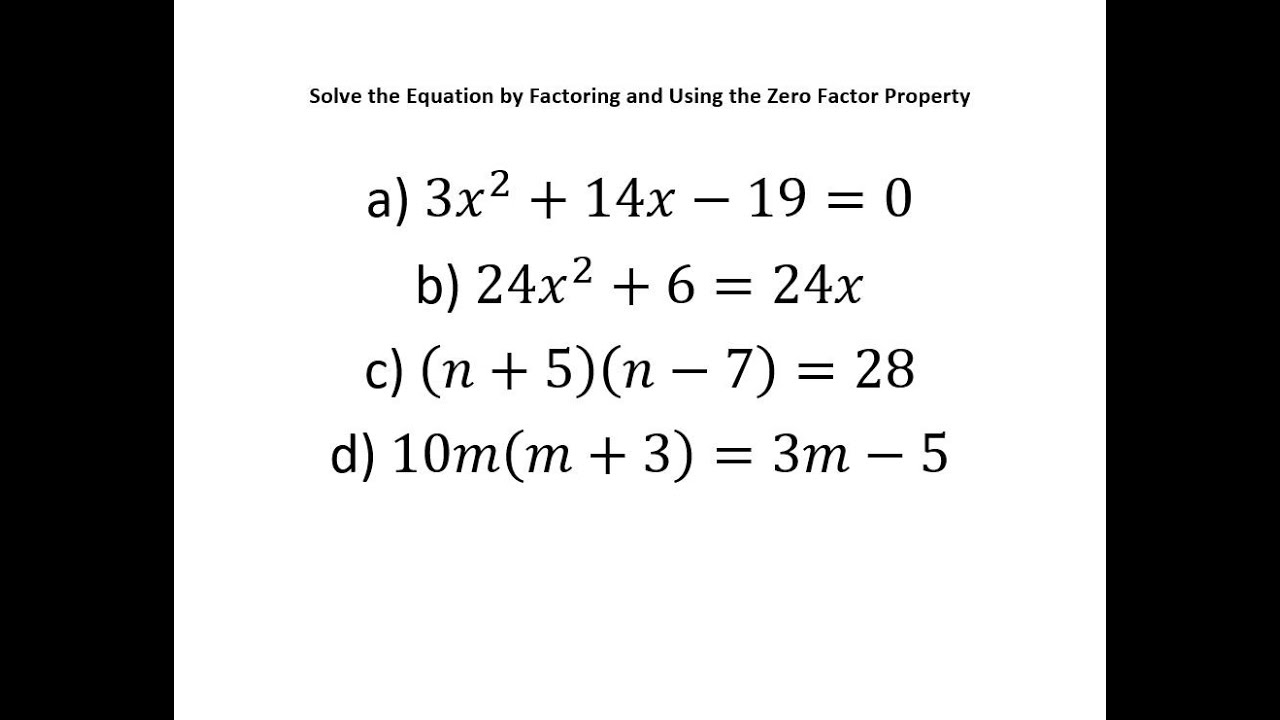The factoring zero product property is a fundamental concept in algebra that provides a powerful tool for solving quadratic equations and understanding polynomial functions. This property states that if the product of two factors equals zero, then at least one of the factors must also equal zero. This principle is crucial for students and anyone working with algebra, as it simplifies the process of finding the roots of equations. By applying this property, we can break down complex equations into simpler parts, making it easier to solve for unknown variables.
In mathematics, particularly in algebra, the ability to factor expressions and understand their implications is essential. The factoring zero product property is central to this understanding, as it allows us to set up equations that can be solved systematically. This property not only aids in solving equations but also helps in graphing polynomial functions and comprehending their behavior. Without a solid grasp of this concept, students may struggle with more advanced topics in algebra and calculus.
In this article, we will delve deeper into the factoring zero product property, exploring its applications, examples, and importance in mathematics. We will cover various aspects, including its definition, how to apply it, and common mistakes to avoid. Whether you are a student looking to improve your algebra skills or a teacher seeking to explain this concept effectively, this guide will provide valuable insights.
What is the Factoring Zero Product Property?
The factoring zero product property can be defined as follows: If a product of two factors is equal to zero (i.e., \(ab = 0\)), then at least one of the factors must be zero (\(a = 0\) or \(b = 0\)). This property is particularly useful when solving quadratic equations of the form \(ax^2 + bx + c = 0\). By factoring the quadratic expression into two binomial expressions, we can apply the zero product property to find the values of \(x\) that satisfy the equation.
How Do You Use the Factoring Zero Product Property?
To apply the factoring zero product property, follow these steps:
- Start with a quadratic equation set equal to zero.
- Factor the quadratic expression into two binomials.
- Set each binomial equal to zero.
- Solve for the variable.
For example, consider the equation \(x^2 - 5x + 6 = 0\). This can be factored into \((x - 2)(x - 3) = 0\). By applying the zero product property, we set each factor equal to zero:
- x - 2 = 0 which gives x = 2
- x - 3 = 0 which gives x = 3
Thus, the solutions are \(x = 2\) and \(x = 3\).
Why is the Factoring Zero Product Property Important?
The factoring zero product property is important for several reasons:
- It simplifies the process of solving quadratic equations.
- It provides a systematic approach to finding roots of polynomials.
- It lays the groundwork for understanding more complex algebraic concepts.
- It aids in graphing polynomial functions and analyzing their behavior.
Common Mistakes When Using the Factoring Zero Product Property?
While applying the factoring zero product property, students may encounter several common mistakes:
- Failing to set the equation equal to zero before factoring.
- Incorrectly factoring the quadratic expression.
- Overlooking extraneous solutions that do not satisfy the original equation.
- Not checking the solutions in the original equation.
What Are Some Examples of the Factoring Zero Product Property?
Here are a few examples of the factoring zero product property in action:
- Example 1: Solve \(x^2 - 7x + 10 = 0\).
- Factor: \((x - 5)(x - 2) = 0\).
- Set each factor equal to zero: \(x - 5 = 0\) or \(x - 2 = 0\).
- Solutions: \(x = 5\) and \(x = 2\).
- Example 2: Solve \(2x^2 + 8x = 0\).
- Factor: \(2x(x + 4) = 0\).
- Set each factor equal to zero: \(2x = 0\) or \(x + 4 = 0\).
- Solutions: \(x = 0\) and \(x = -4\).
How Can You Practice the Factoring Zero Product Property?
To master the factoring zero product property, practice is essential. Here are some strategies:
- Work through example problems in textbooks or online resources.
- Join study groups to discuss and solve problems collaboratively.
- Use educational apps that focus on algebraic concepts.
- Seek help from teachers or tutors when struggling with particular problems.
Conclusion: Mastering the Factoring Zero Product Property
In conclusion, the factoring zero product property is a vital concept in algebra that simplifies the process of solving quadratic equations. By understanding and applying this property, students can unlock the mysteries of polynomial functions and gain confidence in their mathematical abilities. With practice and a clear understanding of how to use this property, anyone can become proficient in algebra and prepare for more advanced mathematical topics.
Article Recommendations
- Amy Allan
- Ixora Maui Yellow
- Napoleon A Concise Biography
- 3 Way Wiring Diagram
- Glenn Plummer
- Free Attractions In Niagara Falls
- Cars With Great Audio Systems
- Lisa Raye Height
- Claudia Gerini
- Morgan Kay Beamer